Physical Review Letters
“Preparation of Matrix Product States with Log-Depth Quantum Circuits”
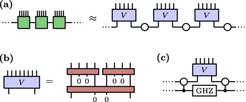
We consider the preparation of matrix product states (MPS) on quantum devices via quantum circuits of local gates. We first prove that faithfully preparing translation-invariant normal MPS of 𝑁 sites requires a circuit depth 𝑇 =Ω(log𝑁). We then introduce an algorithm based on the renormalization-group transformation to prepare normal MPS with an error 𝜀 in depth 𝑇=𝑂[log(𝑁/𝜀)], which is optimal. We also show that measurement and feedback leads to an exponential speedup of the algorithm to 𝑇=𝑂[loglog(𝑁/𝜀)]. Measurements also allow one to prepare arbitrary translation-invariant MPS, including long-range non-normal ones, in the same depth. Finally, the algorithm naturally extends to inhomogeneous MPS.
Access to paper: https://journals.aps.org/prl/abstract/10.1103/PhysRevLett.132.040404












