Physical Review Research
“Thermally induced localization of dopants in a magnetic spin ladder”
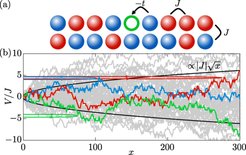
I unveil an emergent localization phenomenon pertaining to the motion of a dopant in a thermal spin lattice. This is rendered localized by thermal spin fluctuations, whereby it is in stark constrast to the intrinsic origin of Anderson localization for quenched disorder. The system of interest consists of spin-1/2 particles organized in a two-leg ladder with nearest-neighbor Ising interactions 𝐽. The motion of a hole—the dopant—is initialized by suddenly removing a spin from the thermal spin ensemble, which then moves along the ladder via nearest-neighbor hopping 𝑡. I find that the hole remains localized for all values of 𝐽/𝑡 and for all nonzero temperatures. The origin is an effective disorder potential seen by the hole and induced by thermal spin fluctuations. Its length scale is found to match with the underlying spin-spin correlation length at low temperatures. For ferromagnetic couplings (𝐽<0), the associated localization length of the hole increases with decreasing temperature and becomes proportional to the correlation length at low temperatures, asymptotically delocalizing at low temperatures. For antiferromagnetic couplings (𝐽>0), there is a smooth crossover between thermal localization at high temperatures to localization driven by the antiferromagnetic order at low temperatures. At infinite temperatures, the dynamics becomes independent of the sign of the spin coupling, whereby the localization length is a universal function of |𝐽|/𝑡, diverging as (𝑡/𝐽)² for |𝐽|≪𝑡. Finally, I analyze a setup with Rydberg-dressed atoms, which naturally realizes finite-range Ising interactions, accessible in current experimental setups. I show that the discovered localization phenomenon can be probed on experimentally accessible length- and timescales, providing a strong testing ground for my predictions.
Access to paper: https://journals.aps.org/prresearch/abstract/10.1103/PhysRevResearch.6.023325?ft=1












